This article is about something I use all the time when I think about poker situations, but I rarely see anyone else talk about it. So even though I’ve written about it before, I think it deserves some more attention.
It’s Bayes theorem. Bayes theorem is one of the most basic ideas in probability theory. If Bayes theorem is new to you, it’s easier to explain how it works than to give its formal definition.
Say you are tested for a symptom-less disease that 1 in 100 people have. The test you take is 95 percent accurate. You test positive. What is the chance you have the disease?
Take a moment to think of your answer.
The answer most people give is 95 percent. This is not correct—and as you’ll see, it’s not even close. I’ll walk you through the logic.
Say we test 10,000 people. Of these 10,000, 100 have the disease, and 9,900 don’t have the disease.
When the 100 who have the disease are tested, we get 95 positive results and 5 negative results. When the 9,900 who don’t have the disease are tested, we get 495 positive results and 9,405 negative results.
So there are 590 total positive results. Of these positive results, 95 have disease, and 495 don’t. If all you know is that you have tested positive, then your chance of having the disease is 95/590 = 0.161 or about 16 percent.
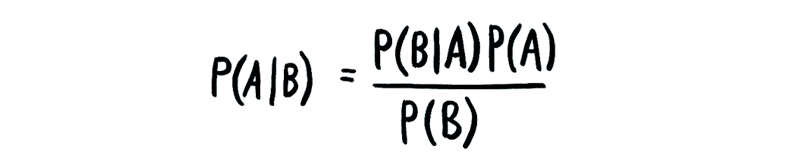
If this idea is new to you, review the example again. You test positive for a disease, and the test is 95 percent accurate. Yet your chance of actually having the disease is only 16 percent.
The reason for this disparity is because of the relative rarity of the disease. Only 1 in 100 people actually have it. But 5 in 100 tested will get the wrong result. Therefore, it’s considerably easier to get the wrong result than it is actually to have the disease.
If we changed the example and the prevalence of the disease dropped to 1 in 1,000, then a 95 percent accurate positive test result would give you only just a couple percent chance of actually having the disease. The rarer the disease is, the more likely it is that the test is wrong rather than that you actually have the disease.
This is a somewhat counterintuitive but extremely powerful concept. For the remainder of the article I’ll share with you a situation where reasoning like this comes into play at the poker table.
Say it’s the river, and your opponent has just bet $500 all-in to an $800 pot. You have a bluff-catcher. If he has the hand he’s representing, you lose. But he could be bluffing. Should you call?
The pot odds say that you need to win 28 percent of the time to justify a call. So that’s how often he needs to be bluffing. If he’s bluffing less often than that, you fold. More often, you call.
There are two relevant pieces of information. First, it’s how likely the player is to bluff when he’s got a busted hand. It’s obvious that this is relevant—but many players stop here when they make this decision. “Is he a bluffer or not? If yes, call. If no, fold.”
But you need another piece of information. How many combinations of the hand he’s repping exist—and what percentage of his total hand range does this encompass.
Say there’s a possible flush on board, and you think your opponent either has the flush or he’s bluffing. You think he would get to the river with 100 total possible hand combinations. Of these, 20 of them are flushes. Another 30 of these combinations are hands like top pair or two pair that he wouldn’t bet, but that he wouldn’t bluff with either. The other 50 combinations are busted, bluff-candidate hands.
He bets $500 into $800. Should you call?
Let’s assume he would bet all 20 flush combinations. You need to win 28 percent of the time to justify a call, so for you to call, your opponent must be bluffing with at least another 8 combinations (because 8/28 = 0.286).
You think he has a total pool of candidate bluffing hands of 50 combinations. He needs to actually bluff with at least 8 of these. The relevant question is, “How often does this player look at a bluffing candidate hand and actually pull the trigger?” If your answer is “at least 16 percent of the time,” then your opponent will bluff with at least 8 out of the 50 combos, and you can call because you can expect to win at least 8/28 of the time.

I want to pull out the two relevant numbers here. First, we had 28 percent. That number is situation-dependent. That is, whether your opponent is bluffing 28 percent of the time when he bets depends on the mix of hands he starts with. If he is gifted with a particularly strong set of hands, he’s unlikely to be bluffing 28 percent of the time because he’s just got so many darn good hands that he’d have to bluff like a madman to get up to 28 percent.
The second number, 16 percent, is purely player-dependent. Is this guy the sort of person who will fire off a big bluff at least 16 percent of the time the opportunity presents itself? A player who bluffs 16 percent of the time will tend to bluff that often whether he’s got a good mix of hands or a bad one.
Let’s say that we’re playing $2-$5, and we think our opponent is on the nitty side. You don’t expect him to bluff all-in 16 percent of the time he’s given the opportunity. Instead, you think it’s more like 5 percent. Most of the time he goes down quietly, but every once in a while he stirs up his courage and lets it fly.
This is a player we will rarely want to call. But let’s put him in an extraordinary circumstance. Say you’ve played a pot, and he shoves all-in. This time he’s not repping a flush but instead a rarer hand like a full house. And, realistically, due to card removal and the fact that he wouldn’t have played certain hands, there are only 5 combinations available that he could possibly hold to justify the bet. Let’s say he’s got 45 hands that are too good to bluff, but not good enough to bet for value. And then he’s got 60 bluff-candidate hands.
He bluffs 5 percent of the time, so out of the 60 total bluff-candidate combos, he bluffs only 3 of them.
When he bets, therefore, he holds a strong hand 5/8 times, and he’s bluffing 3/8 times. His total bluffing frequency in this scenario is 37.5%–so you should call his $500 bet into $800. This is true even though he’s a nitty $2-$5 player who rarely bluffs! The reason it’s true is because the good hands he is repping are so rare, he’s actually nearly as likely to be launching one of his slightly-more-rare bluffs.
Just to be clear, circumstances where a player reps only 5 hand combinations are very uncommon. So I’m definitely not saying you should run around calling nits when they make big bets. Just the opposite—usually you should fold. But to make that decision, it’s not enough to know that your opponent is nitty and rarely bluffs. It also matters how common or rare the hand he’s repping is. If it’s sufficiently rare, you might even consider paying off a nit.


At the risk of sounding very nit picky, but in my experience on these paired boards, what happens is that even the Nits are able to value bet half-way decently and actually bet their big over-pairs because they are not scared of the ephemeral 2-pair that haunts their nightmares. This in turn makes them so value heavy that even though they may in fact bluff as much of the few bluffs that they have, we can’t call simply because we are not going to be good 28% of the time.
I guess I’m harping on this point because I can’t think of a situation where even with advanced Bayes analysis like this calling down a Nitty player is correct. Live players just don’t bluff enough for big bets with no outs left to save them (in other words with air on the river) imo.
Great article. It clarified what math should I do exactly when thinking about combos of hands. Thanks 🙂